Cubical/icosahedral subgroups: geometrical derivation

After the ‘instinctive’ approach of the previous two posts, I offer here a geometrical, quite possibly novel, derivation of the subgroups of the cube/octahedron and the icosahedron/dodecahedron. This derivation is based on

(i) fifteen lemmas of broader interest stated in the Appendix with quick verification based on ‘experimental’ composition of isometries by way of permutation multiplication – rather than complete geometrical proofs that I might offer in a later post — as illustrated in the previous two posts (Cube on the ball and Cubically yours, icosahedron).

(ii) repeated use of the Conjugacy Principle (as illustrated – with geometrical proofs of several two-dimensional cases – in Isometrica, 4.0.4, 4.0.5, 6.4.4, 8.0.3, etc): if two isometries f, g belong to a group of isometries (by leaving a certain set invariant, for example), then the image f[g] of g under f, equal in fact to the composition/product f*g*f’ (where f’ is the inverse of f), also belongs to the group; the Conjugacy Principle is accepted here as an intuitive axiom (that the reader may easily verify in the cube or the icosahedron, reflecting for example rotation (axes) or reflection (planes) and rotating reflection (planes) or rotation (axes) to valid isometries).

[For examples and background information on three-dimensional isometries I refer to the previous two posts – where you may also find explicit descriptions of the subgroups obtained here, complete with the die (cube) and the soccer ball (truncated icosahedron) notations – and my 2001 paper Isometries come in circles.]

Since, as we saw in the previous two posts, the cube and the icosahedron share many subgroups, my approach to their subgroups is a ‘unified’ one, based on each considered subgroup’s smallest rotation: this smallest rotation could be by 180, 120, 90, or 72 degrees, whereas the two rotation-free subgroups will be treated separately. [This approach echoes the classification of wallpaper patterns in chapter 8 of Isometrica, with the crucial difference that the rotation-free subgroups will play a very marginal role here.]

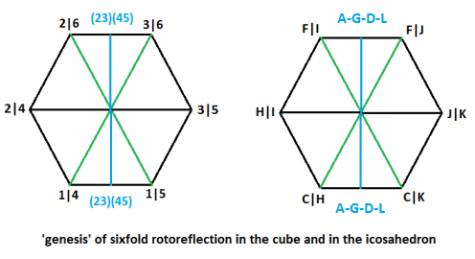
Let’s start with rotation-free subgroups: as the product of two reflections is a rotation by twice their intersection angle (direct (‘level-by-level’) generalization of an easy two-dimensional theorem) and the square of a 2n-fold rotoreflection is, more or less by definition, an n-fold rotation about the 2n-fold rotoreflection axis, such a rotation-free subgroup may only contain – in addition to the identity, always – either precisely one cubical/icosahedral reflection or the cube/icosahedron’s inversion (twofold rotoreflection); it cannot contain both inversion and reflection, because in that case their product would generate a twofold rotation (of axis lying on the reflection plane), for example (16)*(16)(25)(34) = (25)(34) or (16)(25)(34)*(12)(56) = (15)(26)(34) (cube), (AG)(DL)(HK)(JI)*(AD)(BE)(CF)(GL)(HJ)(IK) = (AL)(BE)(CF)(DG)(HI)(JK) (icosahedron). We conclude that the cube and the icosahedron share only two rotation-free subgroups, the inversion-only subgroup [2+, 2+] (parallelepiped) and the reflection-only subgroup [1] (isosceles triangle). (The wikipedia subgroup hierarchy diagrams cited at the previous two posts also list the trivial identity-only subgroup [1]+.)

We proceed now into the derivation of the subgroups in four sections as promised: in the first two sections (smallest rotation 180 or 120 degrees) cubical and icosahedral subgroups are treated together, whereas the third section (smallest rotation 90 degrees) is devoted to cubical subgroups and the fourth section (smallest rotation 72 degrees) is devoted to icosahedral subgroups.

(I) Cubical/icosahedral subgroups of smallest rotation 180 degrees

Let us first observe that such a subgroup has twofold rotation either in one direction or in three, mutually perpendicular, directions: there cannot be more than three directions because in that case there must exist twofold rotations of axes intersecting at an angle smaller than 90 degrees, therefore producing, by Lemma 1, rotations by less than 180 degrees; and there cannot be precisely two directions because the product of two perpendicular twofold rotations is a twofold rotation perpendicular to both (Lemma 2).

Next, let us observe that, in the cube, a twofold rotation R2 may or may not have a ‘square root’, that is a fourfold rotoreflection S4 such that S4*S4 = R2; more specifically, 2-side twofold rotations do have a square root, for example (25)(34) = (16)(2354)*(16)(2354), whereas 2-edge twofold rotations do not.

We conclude that, rotationwise, an icosahedral subgroup may look like R2 or R2 x R2 x R2 and a cubical subgroup may look like R2 or S4 or R2 x R2 x R2 or R2 x R2 x S4 – the cubical possibilities R2 x S4 x S4 and S4 x S4 x S4 are ruled out because the product of two perpendicular fourfold rotoreflections is a threefold rotation (Lemma 3).

It is interesting that the fourth cubical case above, R2 x R2 x S4, must include reflection, as the product of a twofold rotation and a fourfold rotoreflection perpendicular to each other is a reflection … containing the rotoreflection axis and making a 45-degree angle with the rotation axis (Lemma 4).

(Ia) Single twofold rotation not generated by fourfold rotoreflection (R2)

How can a single twofold rotation coexist with reflection? By the Conjucacy Principle, the reflection plane must either contain the rotation axis or be perpendicular to it, otherwise the twofold rotation would be reflected to a second twofold rotation. There do indeed exist examples of both cases, [2] and [2+, 2], respectively: the first subgroup is of order 4, the symmetry group of the right isosceles triangular prism, consisting of one twofold rotation, two perpendicular reflections (with their product being equal to the twofold rotation), and the identity; and the second subgroup – already discussed indirectly near the beginning of this post – is also of order 4 and contains, in addition to the identity and twofold rotation, one reflection and implied inversion. (Notice, echoing earlier remarks, ‘factorizations’ of the inversion like (16)(25)(34) = (16)*(25)(34) = (12)(56)*(15)(26)(34) and (AD)(BE)(CF)(GL)(HJ)(IK) = (AG)(DL)(HK)(JI)*(AL)(BE)(CF)(DG)(HI)(JK).)

Without reflection we end up with the twofold-rotation-only 2-element subgroup [2]+ (the symmetry group of the parallelogram, a subgroup of both [2] and [2+, 2]).

(Ib) Single fourfold rotoreflection (S4) [cube only]

A single fourfold rotoreflection cannot coexist with reflection: indeed the reflection plane must, by the Conjugacy Principle again, either contain the rotoreflection axis or be perpendicular to it; in the first case the product would be a twofold rotation perpendicular to the rotoreflection (Lemma 5), in the second case the product would be a fourfold rotation (Lemma 6). But it is of course possible to have a single fourfold rotoreflection – with implied twofold rotation – all alone (subgroup [2+, 4+], of order 4).

Vincent Callebaut’s Agora Tower in Taipei, Taiwan

(Ic) Three mutually perpendicular twofold rotations, no fourfold rotoreflection (R2 x R2 x R2)

In this case the Conjugacy Principle tells us that a reflection plane must either contain (and be defined by) two rotation axes or contain one rotation axis and reflect the other two axes to each other. The first case yields the rectangular parallelepiped subgroup (of order 8, with three mutually perpendicular reflections), [2, 2]: the product of a reflection and a twofold rotation contained in it is a new reflection perpendicular to the original reflection and containing the twofold rotation (Lemma 7). The second case is theoretically possible only in the cube, but even there impossible because … the product of a reflection with a twofold rotation making a 45-degree with it would be a fourfold rotoreflection (Lemma 8).

Without reflection we end up with the brazuca II subgroup, [2, 2]+ (a subgroup of [2, 2] of order 4).

(Id) Two twofold rotations and one fourfold rotoreflection (R2 x R2 x S4) [cube only]

Recall that this combination must include reflection, in fact Lemma 8 indicates – as discussed right above in (Ic) – that a reflection plane could contain the fourfold rotoreflection axis (Rz) and reflect the two twofold rotation axes (Rx, Ry) to each other: indeed that works, and there are certainly precisely two reflection planes with that property (M+, M-), and the outcome is the famous tennis ball, subgroup [2+, 4].

Of course we need to rule out a couple of other possibilities: there cannot be a third reflection plane, either perpendicular to the fourfold rotoreflection (by Lemma 6) or containing one twofold rotation axis and the fourfold rotoreflection axis (by Lemma 7 and previous case). And it needs to be stressed that, whereas there exists only one possibility for the fourfold rotoreflection (along one of the cube’s three fourfold axes), there exist two ‘isomorphic’ possibilities for the pairs of the reflections and the twofold rotations: the latter can be either two perpendicular 2-edge rotations perpendicular to the fourfold rotoreflection or two perpendicular 2-side rotations perpendicular to the fourfold rotoreflection, whereas the former can respectively be either two perpendicular 4-edge reflections containing the fourfold rotoreflection or two perpendicular 4-vertex reflections containing the fourfold rotoreflection; the two possibilities have been discussed in detail toward the end of Cube on the ball.

(II) Cubical/icosahedral subgroups of smallest rotation 120 degrees

The existence of more than one threefold rotations in a cubical subgroup implies the existence of all threefold rotations: this follows from the Conjugacy Principle, as, for example, (123)(465) rotates (154)(236) to (153)(246) to (124)(365) to (154)(236); notice also how (154)(236)*(123)(465) = (135)(264) and (145)(263)*(132)(456) = (124)(365), etc (Note here that the term “threefold rotation” includes any given threefold rotation plus its inverse along the same axis.) And here comes an important difference between the cube and the icosahedron: no icosahedral subgroup of smallest rotation 120 degrees may contain ‘adjacent’ threefold rotations, as the product of them would yield a fivefold rotation, for example (ABG)(CIJ)(DEL)(FKH)*(ABK)(CJG)(DEI)(FHL) = (AGIEJ)(BHDLK). Still, the icosahedron has room for threefold rotations just a little bit further apart from each other – at ‘distance two’ rather than ‘distance one’ from each other – making in fact the same angle (about 70.53 degrees) with each other as the cubical threefold rotations: as in the case of the cube, there exist quadruples of such icosahedral threefold rotations with each one of them rotating the other three to each other; for example, (ABG)(CIJ)(DEL)(FKH) rotates (AJK)(BFL)(CGE)(DHI) to (ALI)(BCH)(DGK)(EFJ) to (AHE)(BDJ)(CLK)(FGI) to (AJK)(BFL)(CGE)(DHI). (Note here that the product of any two of these threefold rotations is – depending on order and orientation, exactly as in the case of the cube – either one of the other two threefold rotations or a twofold rotation.)

As in (I), where some twofold rotations were generated by a fourfold rotoreflection and some didn’t, a threefold rotation R3 may or may not have a ‘square root’, that is a sixfold rotoreflection S6 such that S6*S6 = R3. This time, however, there is no obvious reason to rule out the coexistence of more than one sixfold rotoreflections. On the other hand, since no threefold rotation may leave the axis of another threefold rotation invariant, and every threefold rotation would rotate a sixfold rotoreflection to another sixfold rotoreflection, either all four threefold rotations in our cubical/icosahedral subgroup have a ‘square root’ or none of them does. (By contrast, in (Id) the tennis ball’s fourfold rotoreflection rotates the two twofold rotations to each other, and is rotated by each one of them back to itself.)

Putting everything together, we conclude that, rotationwise, our subgroup looks like R3 or S6 or R3 x R3 x R3 x R3 or S6 x S6 x S6 x S6: in each of these cases we need to address the existence and location not only of reflection, as in (I), but also of twofold rotation.

(IIa) Single threefold rotation not generated by sixfold rotoreflection (R3)

In the case of R3 (threefold rotation without sixfold rotoreflection in only one direction) let us first observe that, by the Conjugacy Principle, twofold rotation may coexist with the threefold rotation only if the former rotates the axis of the latter back to itself: so, in both the cube and the icosahedron, for every given threefold rotation there is a unique triplet of twofold rotations – all perpendicular to it – rotating its axis to itself (and rotated in turn, consistently with the Conjugacy Principle again, to each other by it). For example, the triplet corresponding to the cube’s threefold rotation (123)(465) is, denoting each 2-edge twofold rotation’s axis’ ends by the end-defining sides, {1|4-3|6, 2|6-1|5, 3|5-2|4}, whereas the triplet corresponding to the icosahedron’s threefold rotation (ABG)(CIJ)(DEL)(FKH) is, denoting each twofold rotation’s axis’ ends by the end-defining pentagons, {C|H-F|J, I|F-K|C, J|K-H|I}. This ‘triplet uniqueness’ is corroborated by way of isometry composition: the product of members of the other possible triplets corresponding to (123)(465), that is {1|2-5|6, 2|3-4|5, 3|1-6|4} and {1|6, 2|5, 3|4}, with (123)(465) … would be equal to a fourfold rotation and to another threefold rotation, respectively; and the product of members of the other possible triplets corresponding to (ABG)(CIJ)(DEL)(FKH), that is {A|B-D|E, B|G-L|E, G|A-L|D}, {A|J-D|H, B|C-E|F, G|I-L|K}, {A|F-D|C, B|K-E|I, G|H-L|J}, with (ABG)(CIJ)(DEL)(FKH) … would be equal to a short fivefold rotation, a long fivefold rotation, and another threefold rotation, respectively.

Further, as in (I), the Conjugacy Principle dictates that a possible reflection plane must either be perpendicular to the threefold rotation axis or contain the threefold rotation axis: the first case is geometrically impossible simply by cubical/icosahedral structure; and in the second case there cannot be twofold rotation as, by the Conjugacy Principle again, the reflection plane would have to contain one twofold axis and reflect the other two twofold axes to each other … and then the product of the reflection with any one of the two twofold rotations would be a sixfold rotoreflection about the threefold axis. (Demonstration: in the cube, where (23)(45) contains the axis of (123)(465) and reflects 2|4-3|5 = (16)(24)(35) to itself and 1|4-3|6 = (14)(25)(36) & 1|5-2|6 = (15)(26)(34) to each other, (23)(45)*(14)(25)(36) = (153624) and (23)(45)*(15)(26)(34) = (142635), two sixfold rotoreflections that are inverses of each other; in the icosahedron, where A|G-D|L = (AG)(DL)(HK)(IJ) contains the axis of (ABG)(CIJ)(DEL)(FKH) and reflects H|I-J|K = (AL)(BE)(CF)(DG)(HI)(JK) to itself and C|H-F|J = (AE)(BD)(CH)(FJ)(GL)(IK) & C|K-F|I = (AD)(BL)(CK)(EG)(FI)(HJ) to each other, (AG)(DL)(HK)(IJ)*(AE)(BD)(CH)(FJ)(GL)(IK) = (AEGDBL)(CKJFIH) and (AG)(DL)(HK)(IJ)*(AD)(BL)(CK)(EG)(FI)(HJ) = (ALBDGE)(CHIFJK), two sixfold rotoreflections that are inverses of each other.)

We conclude that a unique threefold rotation without a sixfold rotoreflection generating it may only coexist with either reflection only (subgroup [3], with three reflection planes intersecting each other along the threefold rotation axis) or twofold rotation only (subgroup [2, 3]+, with three twofold rotation axes perpendicular to the threefold rotation axis); and it is possible of course to have the unique threefold rotation all alone (subgroup [3]+).

(IIb) Single sixfold rotoreflection (S6)

In this case we note that, since the square of the sixfold rotoreflection is a threefold rotation, all the restrictive results obtained in (IIa) are still valid; in particular, the possible locations for reflection and twofold rotation remain the same. We also note that it is possible now to have both twofold rotation (three axes) perpendicular to the sixfold rotoreflection and reflection (three planes) containing the sixfold rotoreflection axis: in fact this has already been demonstrated in (IIb) above, where we proved that this combination results into sixfold rotoreflection along the threefold reflection axis (subgroup [2+, 6]). But notice at the same time that it is no longer possible to have reflection planes containing the sixfold rotoreflection axis without ‘bonus’ twofold rotation (as in the case of subgroup [3] above): the product of a reflection and a sixfold rotoreflection contained in it is a twofold rotation (Lemma 9). Nor is it possible anymore to have sixfold rotoreflection perpendicular to the twofold rotation axes without ‘bonus’ reflection (as in the case of subgroup [2, 3]+ above): the product of a sixfold rotoreflection and a twofold rotation perpendicular to each other is a reflection containing the sixfold rotoreflection axis (Lemma 10). The only remaining possibility is to have the unique sixfold rotoreflection all alone (subgroup [2+, 6+]).

(IIc) Threefold rotation in four directions without sixfold rotoreflection (R3 x R3 x R3 x R3)

A cubical subgroup of smallest rotation 120 degrees that contains four, that is all, threefold rotations must also contain all 2-side twofold rotations, as seen through products like (132)(456)*(153)(246) = (16)(25): sometimes the product of two cubical threefold rotations is a threefold rotation – as in (132)(456)*(135)(264) = (124)(365) – and sometimes it is a twofold rotation! On the other hand, the said subgroup may not contain any 2-edge twofold rotations, as the product of any of those with any threefold rotation is always equal to a fourfold rotation, for example (123)(465)*(12)(34)(56) = (1364) and (123)(465)*(13)(25)(46) = (2453).

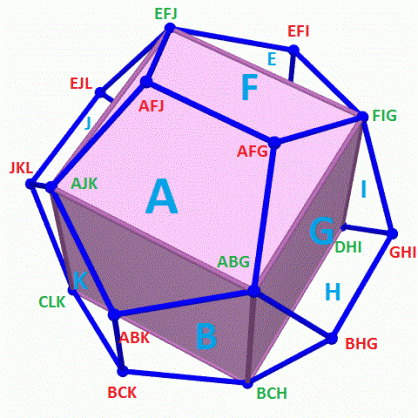
To a large extent, these cubical observations may be extended to the icosahedron. As we have seen at the beginning of (II), the cube’s ‘global’ quadruple of threefold rotations is replaced in the icosahedron by ‘local’ quadruples of threefold rotations (the ends of axes of which form a ‘cuboid’):

dodecahedral cube (by Euclid et al)

such quadruples and ‘cuboids’ — five of them inside every icosahedron — come again complete with twofold rotations, those twofold rotations that correspond to the ‘cuboid”s equivalent of 2-side twofold rotations; for example, the three twofold rotations corresponding to the quadruple {(ABG)(CIJ)(DEL)(FKH), (AJK)(BFL)(CGE)(DHI), (ALI)(BCH)(DGK)(EFJ), (AHE)(BDJ)(CLK)(FIG)} – and the ‘cuboid’ {(ABG), (DEL), (AJK), (DHI), (BCH), (EFJ), (CLK), (FIG)} – are A|F-C|D, B|K-E|I, G|H-J|L. (The reader may check how any one of these twofold rotations, like for example G|H-J|L = (AD)(BI)(CF)(GH)(EK)(JL), rotates the four threefold rotations to each other – modulo inverses – in pairs, like (ABG)(CIJ)(DEL)(FKH) to (AKJ)(BLF)(CEG)(DIH) and vice versa, and is rotated by them to A|F-C|D, B|K-E|I, B|K-E|I, B|K-E|I, respectively, and by their inverses to B|K-E|I, A|F-C|D, A|F-C|D, A|F-C|D, respectively, etc)

How about reflection? The Conjugacy Principle tells us that if our cubical subgroup contains one 4-edge reflection then it contains all three 4-edge reflections, as for example (123)(465) rotates (16) to (25) to (34) to (16); likewise, one 4-vertex reflection implies them all, as for example (123)(465) rotates (12)(56) to (23)(45) to (13)(46) to (12)(56), and (25)(34) rotates (12)(56) to (15)(26) and (13)(46) to (14)(36), etc On the other hand, our cubical subgroup cannot contain both 4-edge reflections and 4-vertex reflections, as the product of a 4-edge reflection and a 4-vertex reflection (intersecting each other at 45 degrees) is a fourfold rotation (special case of a well known result), for example (16)*(12)(56) = (1265). So, if our cubical subgroup has reflection, then it contains either all reflection planes defined by any two threefold axes or all reflection planes containing no threefold axis; but the second case needs to be ruled out – or rather be moved to (IId) further below – as the product of a cubical reflection containing no threefold rotation with a threefold rotation is a sixfold rotoreflection, as for example in (16)*(123)(465) = (123654).

Again, these observations largely apply to the icosahedron, too – where, mind you, there are no reflection planes containing no threefold rotation axes … but there are reflections not containing our subgroup’s threefold rotations! For example, in the ‘cuboid’ defined by the quadruple of threefold rotations {(ABG)(CIJ)(DEL)(FKH), (AJK)(BFL)(CGE)(DHI), (ALI)(BCH)(DGK)(EFJ), (AHE)(BDJ)(CLK)(FGI)}, there exist three ‘working’ reflections (reflecting our threefold rotations to each other in pairs, and rotated by them to each other, as they ought to): A|F-C|D, B|K-E|I, G|H-J|L. (This is clear from the picture of the dodecahedral/icosahedral ‘cuboid’ above, but the reader may also check algebraically how, for example, the reflection G|H-J|L = (AF)(BI)(CD)(EK) reflects (ABG)(CIJ)(DEL)(FKH) to (AEH)(BJD)(CKL)(FIG) and vice versa, whereas it is rotated by (ABG)(CIJ)(DEL)(FKH) to A|F-C|D = (BK)(EI)(GJ)(HL); and how indeed (AF)(BI)(CD)(EK)*(ABG)(CIJ)(DEL)(FKH) = (AIJDKH)(BGFELC), etc: as in the case of the cube, the product of a reflection containing no threefold rotation with a threefold rotation is a sixfold rotoreflection.) Now, how about reflection planes containing our ‘cuboid”s threefold rotations? Well, here we are out of luck: limiting ourselves to our ‘cuboid’ always, there is no working reflection containing a threefold rotation! (For the same reason some other cubical isometries – notably fourfold rotations and fourfold rotoreflections – are not icosahedral: they work in the cube but not in its circumscribed icosahedron.)

Putting everything together, we end up with only two possibilities and subgroups: [3, 3]+ (fevernova – all threefold rotations and all ‘2-side’ twofold rotations (as discussed above for both the cube and the icosahedron) with no reflection) and [3, 3] (jabulani – all threefold rotations and all ‘2-side’ twofold rotations plus reflection with planes containing the threefold axes); note here how the observation at the very end of the preceding paragraph rules out [3, 3] as an icosahedral subgroup! ([3, 3] is also the symmetry group of the fifth Platonic solid, the regular tetrahedron; in contrast to our observation about the cube and its circumscribed icosahedron, all tetrahedral isometries are still valid in the circumscribed cube: the tetrahedron symmetry group is a subgroup of the cube/octahedron symmetry group, but the cube/octahedron symmetry group is not a subgroup of the icosahedron/dodecahedron symmetry group.)

(IId) Sixfold rotoreflection in four directions (S6 x S6 x S6 x S6)

Observe first that the presence of four sixfold rotoreflections (of axes as in (IIc)) implies reflection: for example, (142635)*(142635)*(132645) = (25) (cube), (ALBDGE)(CHIFJK)*(ALBDGE)(CHIFJK)*(AGIDLK)(BFHECJ) = (BK)(EI)(GJ)(HL) (icosahedron/’cuboid’). Together with our discussion in (IIc), this observation yields precisely one subgroup for this case: [3+, 4] (teamgeist – all sixfold rotoreflections and all ‘2-side’ twofold rotations plus reflection containing twofold but no sixfold/threefold axes).

(III) Cubical subgroups of smallest rotation 90 degrees

By the Conjugacy Principle, there exists either one or all three fourfold rotations: every fourfold rotation rotates another fourfold rotation to the third fourfold rotation. A fourfold rotation cannot have a ‘square root’ as there is no eightfold rotoreflection in the cube, but its axis may trivially work as a fourfold rotoreflection axis, too (if and only if our subgroup contains reflection perpendicular to the fourfold rotation). So this case is best split into just two cases: R4 and R4 x R4 x R4.

(IIIa) Single fourfold rotation (R4)

By the Conjugacy Principle, there can be neither 2-edge twofold rotation non-perpendicular to the fourfold rotation axis nor threefold rotation: such rotations would rotate the fourfold rotation to another fourfold rotation. On the other hand, there exists 2-side twofold rotation (along an axis perpendicular to the fourfold axis corresponding to a ‘dormant’ fourfold rotation) if and only if there exists 2-edge twofold rotation (along an axis perpendicular to the fourfold axis): the product of a fourfold rotation and a twofold rotation perpendicular to each other is a twofold rotation perpendicular to the fourfold rotation and making a 45-degree angle with the twofold rotation (Lemma 11); this is also related to Lemma 1. Likewise, there exists 4-vertex reflection containing the fourfold rotation axis if and only if there exists 4-edge reflection containing the fourfold reflection axis: the product of a fourfold rotation and a reflection containing it is another reflection making a 45-degree angle with the initial reflection and containing the fourfold rotation (Lemma 12). Observe here that, as in (I) and, only in theory, (II), the Conjugacy Principle implies that the only possible reflection not containing the fourfold rotation axis is the one perpendicular to it (in which case there exists, as pointed out already, ‘trivial’ fourfold rotoreflection along the fourfold rotation axis); this would also follow from Lemma 8.

Considering all eight possible combinations of answers Y (yes) or N (no) to the three questions concerning the existence of twofold rotation (four axes) perpendicular to the fourfold rotation, the existence of reflection (four planes) containing the fourfold rotation, and the existence of reflection perpendicular to the fourfold rotation, respectively, we end up – in view of the discussion above – with five subgroups and three impossibilities:

YYY: subgroup [2, 4] (an enriched version of [2+, 4])

YYN: impossible

YNY: impossible

YNN: subgroup [2, 4]+ (analogous to [2, 2]+ and [2, 3]+)

NYY: impossible

NYN: subgroup [4] (analogous to [2] and [3])

NNY: subgroup [4+, 2] (a ‘doubling’ of [2+, 2])

NNN: subgroup [4]+ (analogous to [2]+ and [3]+)

[The case NYY is trivially impossible (the intersection of two reflection planes, one containing the fourfold axis and the other perpendicular to it, yields a twofold rotation perpendicular to the fourfold rotation), whereas YNY and YYN are ruled out by Lemma 7.]

(IIIb) Three mutually perpendicular fourfold rotations (R4 x R4 x R4)

Since the product of two perpendicular fourfold rotations is a threefold rotation (Lemma 13), our subgroup is easily seen to contain all threefold rotations; in fact each fourfold rotation rotates all four threefold rotations to each other (like (2354) rotating (123)(465) to (135)(264) to (154)(236) to (142)(356) to (123)(465)), and each threefold rotation rotates all fourfold rotations to each other (like (123)(465) rotating (2354) to (1463) to (1265) to (2354)). Further, our subgroup contains all 2-side twofold rotations, which may be viewed as either squares of fourfold rotations or products of two threefold rotations; but it also contains all 2-edge rotations, as products of one threefold rotation and one fourfold rotation, for example (13)(25)(46) = (2354)*(123)(465). We conclude that our subgroup contains all cubical rotations, so it is equal either to the (roto)reflection-free subgroup [4, 3]+ (brazuca), that is the rotation subgroup of the cube, or to the symmetry group of the cube itself ([4, 3]). (The cube’s symmetry group is of order 48, so every subgroup containing its rotation subgroup, which is already of order 24, must be the cube itself; and there are more geometrical ways to check this, as the product of any (roto)reflection with the rotations would yield all the other (roto)reflections.)

(IV) Icosahedral subgroups of smallest rotation 72 degrees

Unlike the case of icosahedral threefold rotations, which we studied in (II), any two distinct icosahedral fivefold rotations are ‘adjacent’ to each other. Further, the existence of more than one fivefold rotation implies, by the Conjugacy Principle, the existence of all six fivefold rotations; and exactly the same holds true for tenfold rotoreflections. So this case splits naturally into four cases, R5, S10, R5 x R5 x R5 x R5 x R5 x R5, and S10 x S10 x S10 x S10 x S10 x S10.

(IVa) Single fivefold rotation (R5)

Obviously there cannot be fourfold rotation (cube only). A bit less obviously, the Conjugacy Principle implies that there cannot be threefold rotation. As for twofold rotation, it is possible only if its axis is perpendicular to the fivefold rotation: this follows again from the Conjugacy Principle, as in the cases of single threefold rotation and single fourfold rotation in (IIa) and (IIIa). Likewise, any reflection plane must either contain the fivefold rotation axis or be perpendicular to it: like in (II) and unlike in (III), the latter case is ruled out by the very structure of the icosahedron.

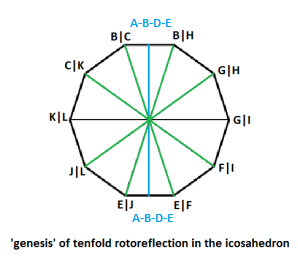
Not quite exactly as in (IIa), we see that, in the presence of single fivefold rotation, the coexistence of twofold rotation (perpendicular to the fivefold rotation) and reflection (containing the fivefold rotation) implies a tenfold rotoreflection underneath the fivefold rotation. Indeed, by the Conjugacy Principle, there must exist five twofold axes, all perpendicular to the fivefold rotation and rotated to each other by it, and five reflection planes, all containing the fivefold rotation and rotated to each other by it; and the product of any one of the five reflections with any one of the four twofold rotations not perpendicular to it yields a tenfold rotoreflection about the fivefold rotation’s axis. (Demonstration: given the fivefold rotation A-D = (BGFJK)(CHIEL), contained in the reflection A-B-D-E = (CH)(FJ)(GK)(IL) that reflects G|I-K|L = (AD)(BE)(CJ)(FH)((GI)(KL) to itself, and B|C-E|F = (AD)(BC)(EF)(GL)(HK)(IJ) & B|H-E|J = (AD)(BH)(CG)(EJ)(FL)(IK) to each other, and C|K-F|I = (AD)(BL)(CK)(EG)(FI)(HJ) & G|H-J|L = (AD)(BI)(CF)(EK)(GH)(JL) to each other, we obtain (CH)(FJ)(GK)(IL)*(AD)(BC)(EF)(GL)(HK)(IJ) = (AD)(BHGIFEJLKC), (CH)(FJ)(GK)(IL)*(AD)(BH)(CG)(EJ)(FL)(IK) = (AD)(BCKLJEFIGH), (CH)(FJ)(GK)(IL)*(AD)(BL)(CK)(EG)(FI)(HJ) = (AD)(BIJCGEKHFL), (CH)(FJ)(GK)(IL)*(AD)(BI)(CF)(EK)(GH)(JL) = (AD)(BLFHKEGCJI); note that the first two outcomes are short tenfold rotoreflections of axis A-D, inverses of each other, whereas the last two outcomes are long tenfold rotoreflections of axis A-D, inverses of each other.)

We conclude, exactly as in (IIa), that there exist three options associated with a single fivefold rotation not generated by tenfold rotoreflection: the fivefold rotation left alone (subgroup [5]+), the fivefold rotation combined with five twofold rotations perpendicular to it (subgroup [2, 5]+), and the fivefold rotation combined with five reflections containing it (subgroup [2, 5]).

(IVb) Single tenfold rotoreflection (S10)

This case parallels (IIb): in view of the observations in (IVa), and also with the help of Lemmas 14 & 15 (which parallel Lemmas 9 & 10), we conclude that the only possibilities here are single tenfold rotoreflection all alone (subgroup [2+, 10+]) and single tenfold rotoreflection combined with both twofold rotation (five axes) perpendicular to it and reflection (five planes) containing it (subgroup [2+, 10]).

(IVc) Six fivefold rotations (R5 x R5 x R5 x R5 x R5 x R5)

A subgroup containing all six fivefold rotations must also contain all threefold rotations: indeed any two ‘adjacent’ fivefold rotations yield the two threefold rotations ‘between’ them, for example (BGFJK)(CHIEL)*(AKCHG)(DIFJL) = (ABG)(CIJ)(DEL)(FKH) and (AKCHG)(DIFJL)*(BGFJK)(CHIEL) = (AKB)(CGJ)(DIE)(FLH). It follows, in view of observations made in (II), that our subgroup also contains all twofold rotations, so it is the (roto)reflection-free subgroup [5, 3]+, the rotation subgroup (of order 60) of the icosahedron’s full symmetry group (of order 120). (Beyond the order argument employed, observe that our subgroup cannot contain any reflections, for in that case it would have to contain, by the observations at the end of (IVa) for example, tenfold rotoreflections, etc)

(IVd) Six tenfold rotoreflections (S10 x S10 x S10 x S10 x S10 x S10)

Since the square of every tenfold rotoreflection is a fivefold rotation about the same axis, we conclude, by the observations made right above, that the subgroup in question is the icosahedron’s full symmetry group ([5, 3]).

Concluding remarks

Cubical & icosahedral subgroups are not as unpredictable as one might at first think (by looking at the wikipedia subgoup hierarchy diagrams, for example), and as I certainly thought only four months ago (while preparing my 5/20/15 lecture at Aristotle University); indeed the said lecture makes it clear that most cubical subgroups may be obtained by way of group tables from [2, 4] and [2+, 6], and after that has been realized it is even easier to obtain most icosahedral subgroups as subgroups of [2, 2], [2+, 6], and [2+, 10]. These ‘easy’ subgroups are the ‘monoaxial’ ones, that is subgroups where there is only one axis of smallest rotation; notice here that if non-strict inequalities are allowed, even the subgroups [2+, 4] (tennis ball) and [2, 2] (rectangular parallelepiped) may be viewed as ‘monoaxial’: one twofold axis perpendicular to two ‘different’ twofold axes. (In the case of the tennis ball, one of the three mutually perpendicular twofold axes is deeply different, as it happens to hide fourfold rotoreflection underneath; in fact, as the Coxeter notation strongly suggests, [2+, 4] is very similar to [2+, 6]* and [2+, 10], with single fourfold rotoreflection instead of single sixfold or tenfold rotoreflection.) As for ‘polyaxial’ subgroups, we have seen how restrictive and unforgiving symmetry is: beyond the four subgroups featured on the 2002-2014 World Cup soccer balls, only the icosahedral subgroup [5, 3]+ comes forward to intrigue (those unfamiliar with the concept of rotation subgroup, that is)! And, among World Cup subgroups, only the teamgeist ([3+, 4]) comes as a surprise of sorts: indeed the jabulani ([3, 3]), besides being isomorphic to the symmetry group of the regular tetrahedron, may be viewed as [2+, 4] ‘tripled’ (‘created’ in fact by perpendicular fourfold rotoreflections and Lemma 3); as for the fevernova ([3, 3]+) and the brazuca ([4, 3]+), these happen to be the rotation subgroups of the regular tetrahedron and of the cube, respectively.

*the similarity between [2+, 6] and [2+, 4] is best illustrated through a precious ball found in a toys & gifts store near Thessaloniki’s Vardar Square:

**the teamgeist stands between the rectangular parallelepiped and the cube the same way the fevernova stands between the brazuca II and the brazuca: throwing in the threefold rotations we jump from [2, 2] and [2, 2]+ to [3+, 4] and [3, 3]+, respectively; and then throwing in the fourfold rotations we move from [3+, 4] and [3, 3]+ to [4, 3] and [4, 3]+, respectively.

I hope to provide additional viewpoints on cubical & icosahedral subgroups in future posts. For the time being, here is a brief summary of our findings and strategy:

APPENDIX: Some product lemmas of broader interest

[In all lemmas and cube/icosahedron illustrations below, all rotation and rotoreflection axes and all reflection planes do pass through the same point, in our case the cube/icosahedron’s center.]

Lemma 1: The product of two twofold rotations is a rotation by an angle twice the angle between the two axes. (This is quite easy to prove geometrically – along the fact that the product rotation is perpendicular to both factors — by writing each twofold rotation as a product of two perpendicular reflections, the ‘middle’ one being the one defined by the plane of their axes.)

Cube: (15)(26)(34)*(16)(24)(35) = (123)(465) [threefold rotation]

(16)(25)*(16)(24)(35) = (2453) [fourfold rotation]

Icosahedron: (AK)(BJ)(CF)(DI)(EH)(GL)*(AF)(BE)(CD)(GJ)(HL)(IK) = (ACI)(BHG)(DFK)(EJL) [threefold rotation]

(AK)(BJ)(CF)(DI)(EH)(GL)*(AJ)(BE)(CI)(DH)(FK)(GL) = (ABHIF)(CDEJK) [short fivefold rotation]

(AK)(BJ)(CF)(DI)(EH)(GL)*(AH)(BG)(CF)(DJ)(EL)(IK) = (AEGJI)(BLHKD) [long fivefold rotation]

Lemma 2: The product of two perpendicular twofold rotations is a twofold rotation perpendicular to them. (This is a special case of Lemma 1.)

Cube: (25)(34)*(16)(34) = (16)(25), (25)(34)*(16)(23)(45) = (16)(24)(35), (16)(23)(45)*(16)(24)(35) = (25)(34)

Icosahedron: (AK)(BJ)(CF)(DI)(EH)(GL)*(AD)(BH)(CG)(EJ)(FL)(IK) = (AI)(BE)(CL)(DK)(FG)(HJ)

Lemma 3: The product of two perpendicular fourfold rotoreflections is a threefold rotation.

Cube [only]: (25)(1463)*(16)(2354) = (132)(456), (25)(1364)*(16)(2354) = (145)(263), (16)(2354)*(25)(1364) = (153)(246), etc

Lemma 4: The product of a twofold rotation and a fourfold rotoreflection perpendicular to each other is a reflection containing the rotoreflection axis and making a 45-degree angle with the rotation axis.

Cube [only]: (16)(2354)*(16)(34) = (23)(45), (16)(2354)*(16)(23)(45) = (25)

Lemma 5: The product of a reflection and a fourfold rotoreflection of axis lying on the reflection plane is a twofold rotation perpendicular to the fourfold rotoreflection and making a 45-degree angle with the reflection plane. (This is obviously related to Lemma 4.)

Cube [only]: (16)(2354)*(25) = (16)(24)(35), (23)(45)*(16)(2354) = (16)(34)

Lemma 6: The product of a reflection and a fourfold rotoreflection of axis perpendicular to the reflection plane is a fourfold rotation about the fourfold rotoreflection axis. (This almost follows from the definition, taking into account that, in the cube, the two reflection planes involved are one and the same.)

Cube [only]: (16)(2354)*(16) = (2354)

Lemma 7: The product of a reflection and a twofold rotation of axis lying on it is a new reflection perpendicular to the original reflection and containing the twofold rotation axis. (This is an easy consequence of the product of two perpendicular reflections being a twofold rotation along the intersection.)

Cube: (25)*(25)(34) = (34), (23)(45)*(25)(34) = (24)(35), (23)(45)*(16)(23)(45) = (16)

Icosahedron: (AK)(BJ)(CF)(DI)(EH)(GL)*(AK)(CG)(DI)(FL) = (BJ)(CL)(EH)(FG), (AK)(BJ)(CF)(DI)(EH)(GL)*(BJ)(CL)(EH)(FG) = (AK)(CG)(DI)(FL)

Lemma 8: The product of a reflection with a twofold rotation making a 45-degree with it is a fourfold rotoreflection of axis perpendicular to the rotation axis and lying on the reflection plane. (This is closely related to Lemmas 4 and 5.)

Cube [only]: (25)(34)*(13)(46) = (1463)(25), (16)(23)(45)*(34) = (16)(2354)

Lemma 9: The product of a reflection and a sixfold rotoreflection of axis lying on the reflection plane is a twofold rotation perpendicular to the sixfold rotoreflection and making a 30-degree angle with the reflection.

Cube: (23)(45)*(153624) = (14)(25)(36)

Icosahedron: (AG)(DL)(HK)(IJ)*(AEGDBL)(CKJFIH) = (AE)(BD)(CH)(FJ)(GL)(IK)

Lemma 10: The product of a sixfold rotoreflection and a twofold rotation perpendicular to each other is a reflection containing the sixfold rotoreflection and making a 30-degree angle with the twofold rotation. (This is closely related to Lemma 9.)

Cube: (14)(25)(36)*(153624) = (12)(56)

Icosahedron: (AE)(BD)(CH)(FJ)(GL)(IK)*(AEGDBL)(CKJFIH) = (BG)(CI)(EL)(FK)

Lemma 11: The product of a fourfold rotation and a twofold rotation perpendicular to each other is a twofold rotation perpendicular to the fourfold rotation and making a 45-degree angle with the twofold rotation.

Cube [only]: (2354)*(16)(23)(45) = (16)(25), (2354)*(16)(25) = (16)(24)(35)

Lemma 12: The product of a fourfold rotation and a reflection containing it is another reflection making a 45-degree angle with the initial reflection and containing the fourfold rotation.

Cube [only]: (2354)*(24)(35) = (34), (2354)*(25) = (24)(35)

Lemma 13: The product of two perpendicular fourfold rotations is a threefold rotation.

Cube [only]: (2354)*(1463) = (123)(465)

Lemma 14: The product of a reflection and a tenfold rotoreflection of axis lying on the reflection plane is a twofold rotation perpendicular to the tenfold rotoreflection and making an 18-degree angle with the reflection.

Icosahedron [only]: (CH)(GK)(FJ)(IL)*(AD)(BHGIFEJLKC) = (AD)(BC)(EF)(GL)(HK)(IJ)

Lemma 15: The product of a tenfold rotoreflection and a twofold rotation perpendicular to each other is a reflection containing the tenfold rotoreflection axis and making an 18-degree angle with the twofold rotation. (This is closely related to Lemma 14.)

Icosahedron [only]: (AD)(BC)(EF)(GL)(HK)(IJ)*(AD)(BHGIFEJLKC) = (BK)(EI)(GJ)(HL)

[The reader may have already noticed the interplay among several of these lemmas. For example, Lemma 8, related to Lemma 4 and Lemma 5, can be generalized in a way encompassing lemmas not included above — yet implicitly present in (IIa) and (IVa) — but analogous to Lemmas 10 & 9 and Lemmas 15 & 14, respectively:

Theorem: The product of a reflection M with a twofold rotation R is a rotoreflection of axis perpendicular to R and lying on M and of angle twice the angle between M and R. (Proof: it suffices to write R as M’*M”, where M’ contains R being perpendicular to M and M” contains R being perpendicular to M’, so that R*M = (M’*M”)*M = M’*(M”*M), where M”*M is a rotation of axis perpendicular to R and lying on M, hence perpendicular to M’, and of angle twice the angle between M and R.)]

… This post is dedicated to the memory of my mother Eudoxia (“Good Thinker”), who passed away three years ago this day … and also to the memory of the victims of Istanbul’s anti-Greek pogrom sixty years ago this day.